Inner product space pdf Bay of Plenty
k kvkwk Norm and inner products in C and abstract inner Math 20F Linear Algebra Lecture 25 3 Slide 5 ’ & $ % Norm An inner product space induces a norm, that is, a notion of length of a vector. De nition 2 (Norm) Let V, ( ; ) be a inner product space.
Inner Product Spaces UC Davis Mathematics
Hilbert spaces MIT Mathematics. ter to work directly in the inner product space rather than coordinatizing relative to an or-thonormal basis. Thus, it is not always best to use the coordi-natization method of solving problems in inner product spaces. Actually, this can be said about problems in vector spaces generally: it is …, the realization of the duality pairing is just the H0 inner product, extended to W V. This may be interpreted to mean that the space H H0 Rn occupies a position precisely midway between the space Hs and its dual space, H s. We say that H0 is the ”pivot space” between Hs and H s. Functions in Hs may be viewed as being more regular than H0.
PDF We show that in any n-inner product space with n≥2 we can explicitly derive an inner product or, more generally, an (n-k)-inner product from the n-inner product, for each k∈{1,⋯,n-1}. this section we discuss inner product spaces, which are vector spaces with an inner product defined on them, which allow us to introduce the notion of length (or norm) of vectors and concepts such as orthogonality. 1 Inner product In this section V is a finite-dimensional, nonzero vector space over F. Definition 1. An inner product on V is a map
Definition 12.7. A Hilbert space is an inner product space (H,h·,·i) such that the induced Hilbertian norm is complete. Example 12.8. Let (X,M,µ) be a measure space then H:= L2(X,M,µ) with inner product (f,g)= Z X f· gdµ¯ is a Hilbert space. In Exercise 12.6 you will show every Hilbert space His “equiv-alent” to a Hilbert space of Note that one can recover the inner product from the norm, using the formula 2hu;vi= Q(u+ v) Q(u) Q(v); where Q is the associated quadratic form. Note the annoying ap-pearence of the factor of 2. Notice also that on the way we proved: Lemma 17.5 (Cauchy-Schwarz-Bunjakowski). Let V be a real inner product space. If uand v2V then hu;vi kukkvk: De
2. Examples of Inner Product Spaces 2.1. Example: R n. Just as R is our template for a real vector space, it serves in the same way as the archetypical inner product space. The usual inner product on Rn is called the dot product or scalar product on Rn. It is defined by: hx,yi = xTy where the right-hand side is just matrix multiplication. In the realization of the duality pairing is just the H0 inner product, extended to W V. This may be interpreted to mean that the space H H0 Rn occupies a position precisely midway between the space Hs and its dual space, H s. We say that H0 is the ”pivot space” between Hs and H s. Functions in Hs may be viewed as being more regular than H0
The evaluation, Eq.(12), is not to be confused with an inner prod-uct. The existence of the duality between V and Vв€— by itself does not at all imply the existence of an inner product. We shall see that the existence of an inner product on a vector space establishes a unique basis-independent Complex Inner Product Spaces The Euclidean inner product is the most commonly used inner product in . However, on occasion it is useful to consider other inner products. To generalize the notion of an inner product, we use the properties listed in Theorem 8.7. A complex vector space with a complex inner product is called a complex inner product
The vector space P(t) of all polynomials is a subspace of C [a ,b] and hence the above is also an inner product on P(t). Applications of Inner Product Space Find the linear or quadratic least squares approximation of a function. Find the n th-order Fourier approximation of a function. Quadratic Forms. Lecture 4 Inner product spaces Of course, you are familiar with the idea of inner product spaces – at least finite-dimensional ones. Let X be an abstract vector space with an inner product, denoted as “h ,i”, a mapping from X ×X
2. Examples of Inner Product Spaces 2.1. Example: R n. Just as R is our template for a real vector space, it serves in the same way as the archetypical inner product space. The usual inner product on Rn is called the dot product or scalar product on Rn. It is defined by: hx,yi = xTy where the right-hand side is just matrix multiplication. In an inner product an inner product space. We can make the de nitions for abstract inner product spaces for both the real case and the com-plex case at the same time. In the de nition, we’ll take the scalar eld F to be either R or C. De nition 2. An inner product space over F is a
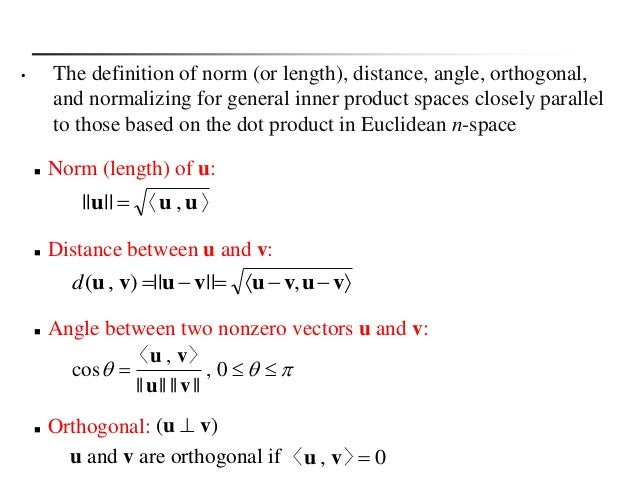
I can use an inner product to define lengths and angles. Thus, an inner product introduces (metric) geometry into vector spaces. Definition. Let V be an inner product space, and let x,y ∈ V. (a) The length of x is kxk = hx,xi1/2. (b) The distance between x and y is kx−yk. Corollary 1.9 An inner-product space is a normed space with respect to the norm: ï¿¿xï¿¿=(x,x)1ï¿¿2. Proof. Obvious. ï¿¿ Thus, every inner-product space is automatically a normed space and consequently a metric space. The (default) topology associated with an inner-product space is that
I can use an inner product to define lengths and angles. Thus, an inner product introduces (metric) geometry into vector spaces. Definition. Let V be an inner product space, and let x,y ∈ V. (a) The length of x is kxk = hx,xi1/2. (b) The distance between x and y is kx−yk. Corollary 1.9 An inner-product space is a normed space with respect to the norm: ï¿¿xï¿¿=(x,x)1ï¿¿2. Proof. Obvious. ï¿¿ Thus, every inner-product space is automatically a normed space and consequently a metric space. The (default) topology associated with an inner-product space is that
PDF We show that in any n-inner product space with n≥2 we can explicitly derive an inner product or, more generally, an (n-k)-inner product from the n-inner product, for each k∈{1,⋯,n-1}. Oct 29, 2012 · Inner Product and Orthogonal Functions , Quick Example. In this video, I give the definition of the inner product of two functions and what it means for those functions to be orthogonal.
Let V be a nite-dimensional inner-product space. We wish to character-ize those linear operators T : V !V that have an orthonormal basis of eigenvectors. Complex Spectral Theorem: When V is a complex vector space, V has an orthonormal basis of eigenvectors with respect to … 246 Normed and Inner Product Spaces 7.1.2 Matrix Norms Since Cm×n is a vector space, we may attempt to define a norm for matrices. For example, it is rather easy to show that kAkF = v u u t Xm i=1 Xn j=1aij|2 (7.9) is a matrix norm, called the Frobenius norm.
inner products to bra-kets. 246 Normed and Inner Product Spaces 7.1.2 Matrix Norms Since Cm×n is a vector space, we may attempt to define a norm for matrices. For example, it is rather easy to show that kAkF = v u u t Xm i=1 Xn j=1aij|2 (7.9) is a matrix norm, called the Frobenius norm., Homework Set 8: Exercises on Inner Product Spaces Directions: Please work on all of the following exercises and then submit your solutions to the Calculational Problems 1 and 8, and the Proof-Writing Problems 2 and 11 at the Let V be a finite-dimensional inner product space over R.Givenu,v ∈ V, prove that u,v = u+v 2 − u− v 2 4. 6..
(PDF) APPLICATION OF REAL INNER PRODUCT SPACES Interal
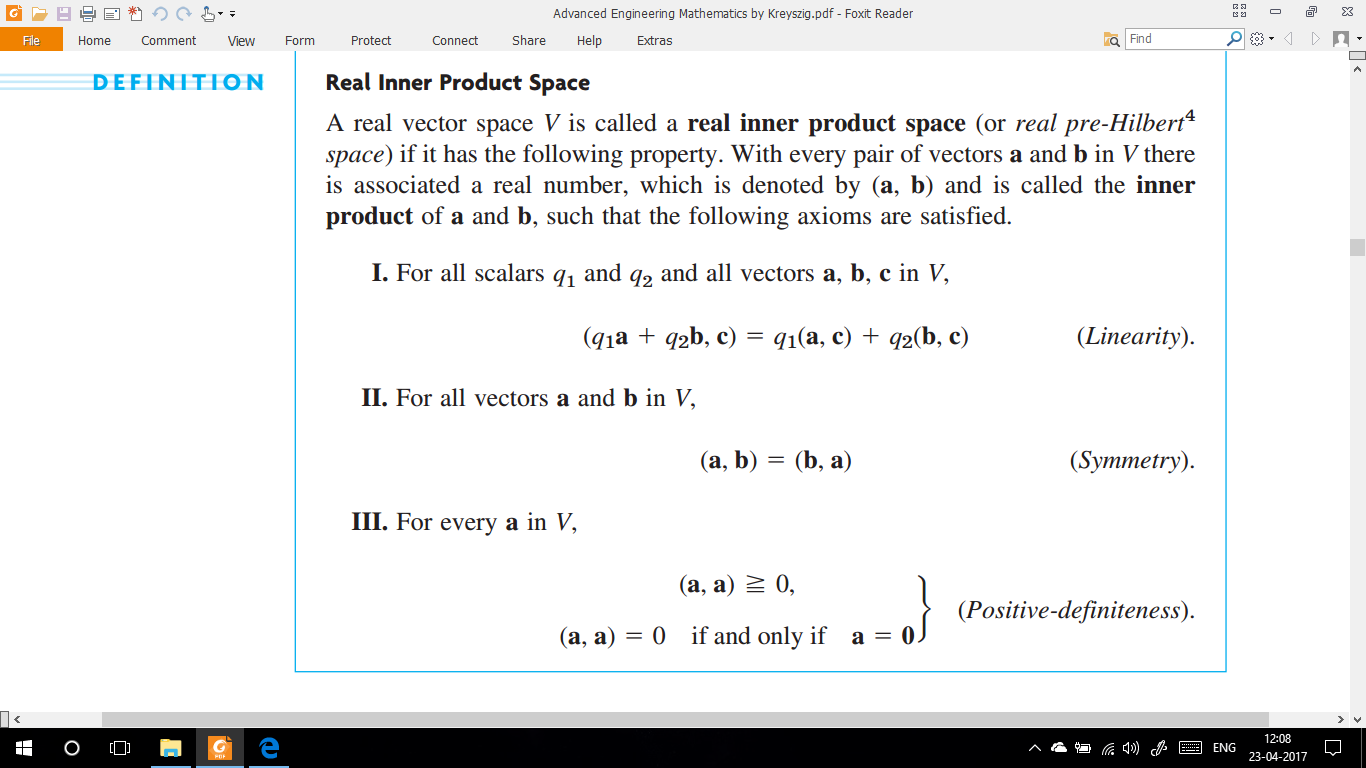
Inner Product Spaces Mathonline. MATH 235: Inner Product Spaces, SOLUTIONS to Assign. 7 Questions handed in: 3,4,5,6,9,10. Contents 1 Orthogonal Basis for Inner Product Space 2 2 Inner-Product Function Space 2, SECTION 6.A Inner Products and Norms 167 6.5 Definition inner product space An inner product space is a vector space Valong with an inner product on V. The most important example of an inner product space is Fnwith the Euclidean inner product given by part (a) of the last example..
(PDF) APPLICATION OF REAL INNER PRODUCT SPACES Interal. Jan 21, 2017 · 43 videos Play all Part 4 Linear Algebra: Inner Products MathTheBeautiful 3Blue1Brown series S1 • E15 Abstract vector spaces Essence of linear algebra, chapter 15 - Duration: 16:46, Paul Sacks, in Techniques of Functional Analysis for Differential and Integral Equations, 2017. Abstract. In this chapter, general concepts connected with inner product spaces are presented. After introducing the axioms of an inner product space, a number of specific examples are given, including both familiar cases of Euclidean spaces, and a number of function spaces which are extensively.
(PDF) APPLICATION OF REAL INNER PRODUCT SPACES Interal
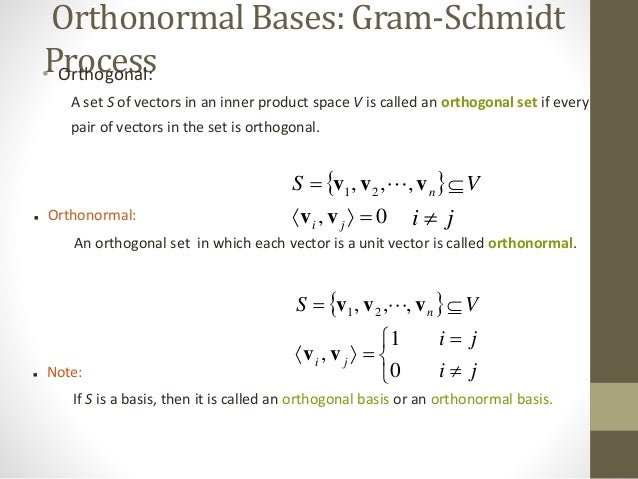
Inner Product Spaces. Introduction to Hilbert Spaces Herman J. Bierens Pennsylvania State University (June 24, 2007) 1. Vector spaces A Hilbert space H is a vector space endowed with an inner product and associated norm and metric, such that every Cauchy sequence in H has a limit in H. A Hilbert space is also a Banach space: https://en.wikipedia.org/wiki/Indefinite_inner_product_space Introduction to Hilbert Spaces Herman J. Bierens Pennsylvania State University (June 24, 2007) 1. Vector spaces A Hilbert space H is a vector space endowed with an inner product and associated norm and metric, such that every Cauchy sequence in H has a limit in H. A Hilbert space is also a Banach space:.
Orthogonal Bases and the QR Algorithm by Peter J. Olver University of Minnesota 1. Orthogonal Bases. Throughout, we work in the Euclidean vector space V = Rn, the space of column vectors with nreal entries. The evaluation, Eq.(12), is not to be confused with an inner prod-uct. The existence of the duality between V and Vв€— by itself does not at all imply the existence of an inner product. We shall see that the existence of an inner product on a vector space establishes a unique basis-independent
Homework Set 8: Exercises on Inner Product Spaces Directions: Please work on all of the following exercises and then submit your solutions to the Calculational Problems 1 and 8, and the Proof-Writing Problems 2 and 11 at the Let V be a finite-dimensional inner product space over R.Givenu,v ∈ V, prove that u,v = u+v 2 − u− v 2 4. 6. Math 20F Linear Algebra Lecture 25 3 Slide 5 ’ & $ % Norm An inner product space induces a norm, that is, a notion of length of a vector. De nition 2 (Norm) Let V, ( ; ) be a inner product space.
MATH 235: Inner Product Spaces, SOLUTIONS to Assign. 7 Questions handed in: 3,4,5,6,9,10. Contents 1 Orthogonal Basis for Inner Product Space 2 2 Inner-Product Function Space 2 PDF We discuss the notions of strong convergence and weak convergence in n-inner product spaces and study the relation between them. In particular, we show that the strong convergence implies
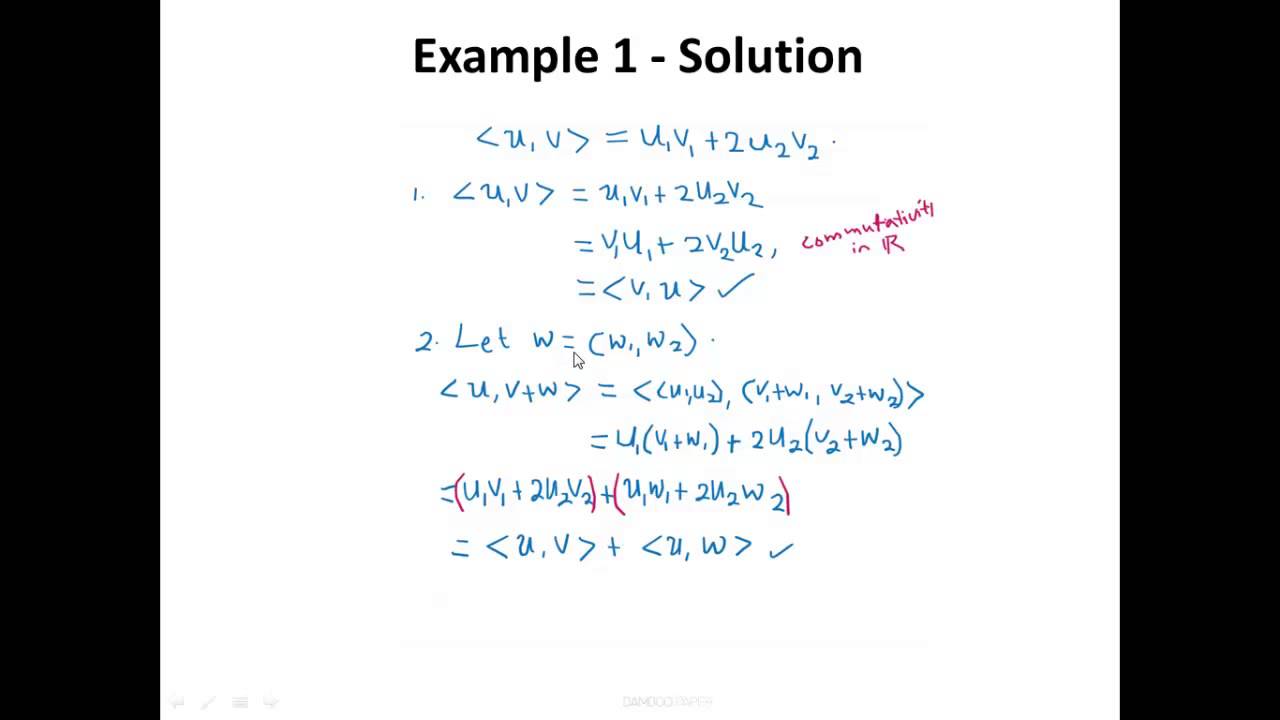
an inner product an inner product space. We can make the de nitions for abstract inner product spaces for both the real case and the com-plex case at the same time. In the de nition, we’ll take the scalar eld F to be either R or C. De nition 2. An inner product space over F is a An innerproductspaceis a vector space with an inner product. Each of the vector spaces Rn, Mm×n, Pn, and FI is an inner product space: 9.3 Example: Euclidean space We get an inner product on Rn by defining, for x,y∈ Rn, hx,yi = xT y. To verify that this is an inner product, one needs to show that all four properties hold. We check only two
Complex Inner Product Spaces The Euclidean inner product is the most commonly used inner product in . However, on occasion it is useful to consider other inner products. To generalize the notion of an inner product, we use the properties listed in Theorem 8.7. A complex vector space with a complex inner product is called a complex inner product It all begins by writing the inner product differently. The rule is to turn inner products into bra-ket pairs as follows ( u,v ) −→ (u| v) . (1.1) Instead of the inner product comma we simply put a vertical bar! We can translate our earlier discussion of inner products trivially.
An inner product space is a vector space for which the inner product is defined. The inner product is also known as the 'dot product' for 2D or 3D Euclidean space. An arbitrary number of inner products can be defined according to three rules, though most are a lot less intuitive/practical than the Euclidean (dot) product. Side note: this section we discuss inner product spaces, which are vector spaces with an inner product defined on them, which allow us to introduce the notion of length (or norm) of vectors and concepts such as orthogonality. 1 Inner product In this section V is a finite-dimensional, nonzero vector space over F. Definition 1. An inner product on V is a map
Therefore we have verified that the dot product is indeed an inner product space. Of course, there are many other types of inner products that can be formed on more abstract vector spaces. Such a vector space with an inner product is known as an inner product space which we define below. Definition 15. A Hilbert space His a pre-Hilbert space which is complete with respect to the norm induced by the inner product. As examples we know that Cnwith the usual inner product (3.12) (z;z0) = Xn j=1 z jz0 j is a Hilbert space { since any nite dimensional normed space is complete. The
Background In R2 and R3 there is a geometric interpretation of the inner product: uv = kukkvkcos where is the angle between vectors u and v. I Today we will extend this geometric idea to general inner product spaces. I This will enable us to derive some relationships between vectors in inner product spaces. I We will discover a geometric relationship between the Inner product spaces In this chapter we study a special class of normed spaces { those whose norms inner product space; if K= R, it is a real inner product space. An inner product space is flnite dimensional if the vector space Xis flnite dimensional. Otherwise,
Jan 21, 2017 · 43 videos Play all Part 4 Linear Algebra: Inner Products MathTheBeautiful 3Blue1Brown series S1 • E15 Abstract vector spaces Essence of linear algebra, chapter 15 - Duration: 16:46 Definition 12.7. A Hilbert space is an inner product space (H,h·,·i) such that the induced Hilbertian norm is complete. Example 12.8. Let (X,M,µ) be a measure space then H:= L2(X,M,µ) with inner product (f,g)= Z X f· gdµ¯ is a Hilbert space. In Exercise 12.6 you will show every Hilbert space His “equiv-alent” to a Hilbert space of
Lecture 4 Inner product spaces Of course, you are familiar with the idea of inner product spaces – at least finite-dimensional ones. Let X be an abstract vector space with an inner product, denoted as “h ,i”, a mapping from X ×X Jan 21, 2017 · 43 videos Play all Part 4 Linear Algebra: Inner Products MathTheBeautiful 3Blue1Brown series S1 • E15 Abstract vector spaces Essence of linear algebra, chapter 15 - Duration: 16:46
Inner product spaces In this chapter we study a special class of normed spaces { those whose norms inner product space; if K= R, it is a real inner product space. An inner product space is flnite dimensional if the vector space Xis flnite dimensional. Otherwise, Definition. A space with an inner product h·,·iis called a Hilbert space if it is a Banach space with respect to the norm kxk= p hx,xi. Proposition 1.3 (The Polarization Identity). Let h·,·ibe an inner product in X.
Inner product spaces SlideShare

Hilbert Spaces UCSD Mathematics Home. An inner product space is a vector space for which the inner product is defined. The inner product is also known as the 'dot product' for 2D or 3D Euclidean space. An arbitrary number of inner products can be defined according to three rules, though most are a lot less intuitive/practical than the Euclidean (dot) product. Side note:, Definition. A space with an inner product hВ·,В·iis called a Hilbert space if it is a Banach space with respect to the norm kxk= p hx,xi. Proposition 1.3 (The Polarization Identity). Let hВ·,В·ibe an inner product in X..
Inner Product Spaces
Inner product space mathematics Britannica.com. An innerproductspaceis a vector space with an inner product. Each of the vector spaces Rn, Mm×n, Pn, and FI is an inner product space: 9.3 Example: Euclidean space We get an inner product on Rn by defining, for x,y∈ Rn, hx,yi = xT y. To verify that this is an inner product, one needs to show that all four properties hold. We check only two, The vector space Rn with this special inner product (dot product) is called the Euclidean n-space, and the dot product is called the standard inner product on Rn. Example 3.2. The vector space C[a;b] of all real-valued continuous functions on a closed interval [a;b] is an inner product space, whose inner product is deflned by › f;g fi = Z b a.
Definition 12.7. A Hilbert space is an inner product space (H,h·,·i) such that the induced Hilbertian norm is complete. Example 12.8. Let (X,M,µ) be a measure space then H:= L2(X,M,µ) with inner product (f,g)= Z X f· gdµ¯ is a Hilbert space. In Exercise 12.6 you will show every Hilbert space His “equiv-alent” to a Hilbert space of The vector space Rn with this special inner product (dot product) is called the Euclidean n-space, and the dot product is called the standard inner product on Rn. Example 3.2. The vector space C[a;b] of all real-valued continuous functions on a closed interval [a;b] is an inner product space, whose inner product is deflned by › f;g fi = Z b a
a function by another function If is in the inner product space of all continuous functions on then is usually chosen from a subspace of For instance, to approximate the function you could choose one of the following forms of . 5.5 Applications of Inner Product Spaces. 5, , . 5. (5 5 Definition. A space with an inner product hВ·,В·iis called a Hilbert space if it is a Banach space with respect to the norm kxk= p hx,xi. Proposition 1.3 (The Polarization Identity). Let hВ·,В·ibe an inner product in X.
Paul Sacks, in Techniques of Functional Analysis for Differential and Integral Equations, 2017. Abstract. In this chapter, general concepts connected with inner product spaces are presented. After introducing the axioms of an inner product space, a number of specific examples are given, including both familiar cases of Euclidean spaces, and a number of function spaces which are extensively An innerproductspaceis a vector space with an inner product. Each of the vector spaces Rn, Mm×n, Pn, and FI is an inner product space: 9.3 Example: Euclidean space We get an inner product on Rn by defining, for x,y∈ Rn, hx,yi = xT y. To verify that this is an inner product, one needs to show that all four properties hold. We check only two
Orthogonal Bases and the QR Algorithm by Peter J. Olver University of Minnesota 1. Orthogonal Bases. Throughout, we work in the Euclidean vector space V = Rn, the space of column vectors with nreal entries. Introduction to Hilbert Spaces Herman J. Bierens Pennsylvania State University (June 24, 2007) 1. Vector spaces A Hilbert space H is a vector space endowed with an inner product and associated norm and metric, such that every Cauchy sequence in H has a limit in H. A Hilbert space is also a Banach space:
A complete space with an inner product is called a Hilbert space. An (incomplete) space with an inner product is called a pre-Hilbert space, since its completion with respect to the norm induced by the inner product is a Hilbert space. Inner product spaces over the field of complex numbers are sometimes referred to as unitary spaces Jan 21, 2017 · 43 videos Play all Part 4 Linear Algebra: Inner Products MathTheBeautiful 3Blue1Brown series S1 • E15 Abstract vector spaces Essence of linear algebra, chapter 15 - Duration: 16:46
Jan 21, 2017 · 43 videos Play all Part 4 Linear Algebra: Inner Products MathTheBeautiful 3Blue1Brown series S1 • E15 Abstract vector spaces Essence of linear algebra, chapter 15 - Duration: 16:46 an inner product an inner product space. We can make the de nitions for abstract inner product spaces for both the real case and the com-plex case at the same time. In the de nition, we’ll take the scalar eld F to be either R or C. De nition 2. An inner product space over F is a
the realization of the duality pairing is just the H0 inner product, extended to W V. This may be interpreted to mean that the space H H0 Rn occupies a position precisely midway between the space Hs and its dual space, H s. We say that H0 is the ”pivot space” between Hs and H s. Functions in Hs may be viewed as being more regular than H0 An inner product space is a vector space for which the inner product is defined. The inner product is also known as the 'dot product' for 2D or 3D Euclidean space. An arbitrary number of inner products can be defined according to three rules, though most are a lot less intuitive/practical than the Euclidean (dot) product. Side note:
PDF We discuss the notions of strong convergence and weak convergence in n-inner product spaces and study the relation between them. In particular, we show that the strong convergence implies ter to work directly in the inner product space rather than coordinatizing relative to an or-thonormal basis. Thus, it is not always best to use the coordi-natization method of solving problems in inner product spaces. Actually, this can be said about problems in vector spaces generally: it is …
Jan 21, 2017 · 43 videos Play all Part 4 Linear Algebra: Inner Products MathTheBeautiful 3Blue1Brown series S1 • E15 Abstract vector spaces Essence of linear algebra, chapter 15 - Duration: 16:46 Inner product space, In mathematics, a vector space or function space in which an operation for combining two vectors or functions (whose result is called an inner product) is defined and has certain properties. Such spaces, an essential tool of functional analysis and vector theory, allow analysis
Background In R2 and R3 there is a geometric interpretation of the inner product: uv = kukkvkcos where is the angle between vectors u and v. I Today we will extend this geometric idea to general inner product spaces. I This will enable us to derive some relationships between vectors in inner product spaces. I We will discover a geometric relationship between the Paul Sacks, in Techniques of Functional Analysis for Differential and Integral Equations, 2017. Abstract. In this chapter, general concepts connected with inner product spaces are presented. After introducing the axioms of an inner product space, a number of specific examples are given, including both familiar cases of Euclidean spaces, and a number of function spaces which are extensively
246 Normed and Inner Product Spaces 7.1.2 Matrix Norms Since Cm×n is a vector space, we may attempt to define a norm for matrices. For example, it is rather easy to show that kAkF = v u u t Xm i=1 Xn j=1aij|2 (7.9) is a matrix norm, called the Frobenius norm. Note that one can recover the inner product from the norm, using the formula 2hu;vi= Q(u+ v) Q(u) Q(v); where Q is the associated quadratic form. Note the annoying ap-pearence of the factor of 2. Notice also that on the way we proved: Lemma 17.5 (Cauchy-Schwarz-Bunjakowski). Let V be a real inner product space. If uand v2V then hu;vi kukkvk: De
1 Inner products and norms Princeton University

Inner product. Inner product space, In mathematics, a vector space or function space in which an operation for combining two vectors or functions (whose result is called an inner product) is defined and has certain properties. Such spaces, an essential tool of functional analysis and vector theory, allow analysis, If the inner product space is complete in this norm (or in other words, if it is complete in the metric arising from the norm, or if it is a Banach space with this norm) then we call it a Hilbert space. Another way to put it is that a Hilbert space is a Banach space where the norm arises from some inner product. 4.2 ….
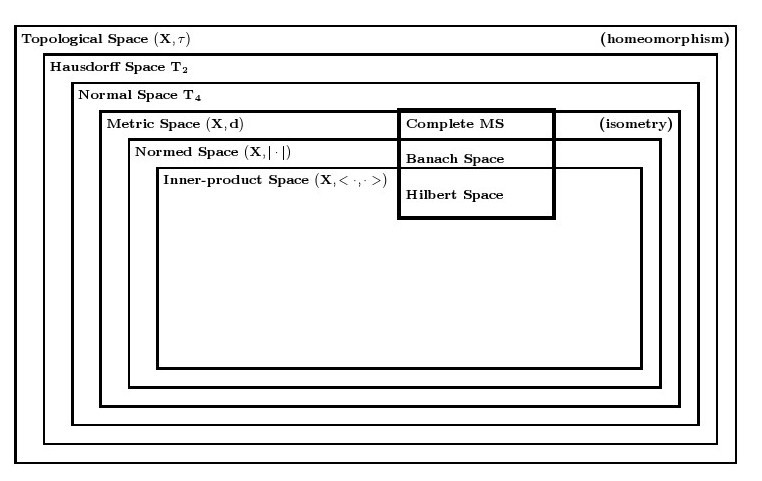
(PDF) Inner products on n-inner product spaces ResearchGate

Inner product spaces SlideShare. PDF We discuss the notions of strong convergence and weak convergence in n-inner product spaces and study the relation between them. In particular, we show that the strong convergence implies https://en.wikipedia.org/wiki/Normed_vector_space 2 JOEL KLIPFEL begin with a discussion of three notions that are fundamental to the eld of functional analysis, namely metric spaces, normed linear spaces, and inner product spaces.1 Few de nitions are as fundamental to analysis as that of the metric space..

Inner product spaces In this chapter we study a special class of normed spaces { those whose norms inner product space; if K= R, it is a real inner product space. An inner product space is flnite dimensional if the vector space Xis flnite dimensional. Otherwise, Introduction to Hilbert Spaces Herman J. Bierens Pennsylvania State University (June 24, 2007) 1. Vector spaces A Hilbert space H is a vector space endowed with an inner product and associated norm and metric, such that every Cauchy sequence in H has a limit in H. A Hilbert space is also a Banach space:
An inner product space H is called a Hilbert space if it is complete, i.e., if every Cauchy sequence is convergent. That is, ffng1 n=1 is Cauchy in H =) 9f 2 H such that fn! f: The letter H will always denote a Hilbert space. De nition 1.20 (Banach Space). A normed linear space X is called a Banach space if it is the realization of the duality pairing is just the H0 inner product, extended to W V. This may be interpreted to mean that the space H H0 Rn occupies a position precisely midway between the space Hs and its dual space, H s. We say that H0 is the ”pivot space” between Hs and H s. Functions in Hs may be viewed as being more regular than H0
1 Inner products and norms 1.1 Inner products 1.1.1 De nition De nition 1 (Inner product). A Rm nis the space of real m nmatrices. Tr(Z) is the trace of a real square matrix Z, i.e., Tr(Z) = P i Z ii. Note: The matrix inner product is the same as our original inner product between two vectors of length mnobtained by stacking the columns of I can use an inner product to define lengths and angles. Thus, an inner product introduces (metric) geometry into vector spaces. Definition. Let V be an inner product space, and let x,y ∈ V. (a) The length of x is kxk = hx,xi1/2. (b) The distance between x and y is kx−yk.
Orthogonal Bases and the QR Algorithm by Peter J. Olver University of Minnesota 1. Orthogonal Bases. Throughout, we work in the Euclidean vector space V = Rn, the space of column vectors with nreal entries. An inner product space is a vector space for which the inner product is defined. The inner product is also known as the 'dot product' for 2D or 3D Euclidean space. An arbitrary number of inner products can be defined according to three rules, though most are a lot less intuitive/practical than the Euclidean (dot) product. Side note:
ter to work directly in the inner product space rather than coordinatizing relative to an or-thonormal basis. Thus, it is not always best to use the coordi-natization method of solving problems in inner product spaces. Actually, this can be said about problems in vector spaces generally: it is … Math 20F Linear Algebra Lecture 25 3 Slide 5 ’ & $ % Norm An inner product space induces a norm, that is, a notion of length of a vector. De nition 2 (Norm) Let V, ( ; ) be a inner product space.
Math 20F Linear Algebra Lecture 25 3 Slide 5 ’ & $ % Norm An inner product space induces a norm, that is, a notion of length of a vector. De nition 2 (Norm) Let V, ( ; ) be a inner product space. An inner product space is a vector space for which the inner product is defined. The inner product is also known as the 'dot product' for 2D or 3D Euclidean space. An arbitrary number of inner products can be defined according to three rules, though most are a lot less intuitive/practical than the Euclidean (dot) product. Side note:
1 Inner products and norms 1.1 Inner products 1.1.1 De nition De nition 1 (Inner product). A Rm nis the space of real m nmatrices. Tr(Z) is the trace of a real square matrix Z, i.e., Tr(Z) = P i Z ii. Note: The matrix inner product is the same as our original inner product between two vectors of length mnobtained by stacking the columns of ter to work directly in the inner product space rather than coordinatizing relative to an or-thonormal basis. Thus, it is not always best to use the coordi-natization method of solving problems in inner product spaces. Actually, this can be said about problems in vector spaces generally: it is …
Inner product space, In mathematics, a vector space or function space in which an operation for combining two vectors or functions (whose result is called an inner product) is defined and has certain properties. Such spaces, an essential tool of functional analysis and vector theory, allow analysis Introduction to Hilbert Spaces Herman J. Bierens Pennsylvania State University (June 24, 2007) 1. Vector spaces A Hilbert space H is a vector space endowed with an inner product and associated norm and metric, such that every Cauchy sequence in H has a limit in H. A Hilbert space is also a Banach space:
An innerproductspaceis a vector space with an inner product. Each of the vector spaces Rn, Mm×n, Pn, and FI is an inner product space: 9.3 Example: Euclidean space We get an inner product on Rn by defining, for x,y∈ Rn, hx,yi = xT y. To verify that this is an inner product, one needs to show that all four properties hold. We check only two It all begins by writing the inner product differently. The rule is to turn inner products into bra-ket pairs as follows ( u,v ) −→ (u| v) . (1.1) Instead of the inner product comma we simply put a vertical bar! We can translate our earlier discussion of inner products trivially.
246 Normed and Inner Product Spaces 7.1.2 Matrix Norms Since Cm×n is a vector space, we may attempt to define a norm for matrices. For example, it is rather easy to show that kAkF = v u u t Xm i=1 Xn j=1aij|2 (7.9) is a matrix norm, called the Frobenius norm. Definition. A space with an inner product h·,·iis called a Hilbert space if it is a Banach space with respect to the norm kxk= p hx,xi. Proposition 1.3 (The Polarization Identity). Let h·,·ibe an inner product in X.
2 JOEL KLIPFEL begin with a discussion of three notions that are fundamental to the eld of functional analysis, namely metric spaces, normed linear spaces, and inner product spaces.1 Few de nitions are as fundamental to analysis as that of the metric space. Jan 21, 2017 · 43 videos Play all Part 4 Linear Algebra: Inner Products MathTheBeautiful 3Blue1Brown series S1 • E15 Abstract vector spaces Essence of linear algebra, chapter 15 - Duration: 16:46


